The Connect Four Challenge, an Introduction

Here is a trail of animation analysis I’ve been exploring, that I’d like to share.

I intend to post it as a series of thoughts, which lead into some potentially useful insights into the basics of timing and spacing, and patterns of keys in the graph editor, accordingly.This posting is the first in the series.
 

The "connect four" challenge

The basic challenge is as follows:

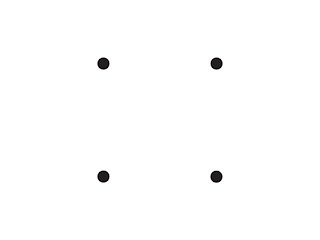
Take a set of four dots in the same pattern as seen on a dice.

Consider each dot to be a key pose in a second-long animated loop.

Connect the dots with keys that are evenly distributed both in timing and spacing.

Simple, right? After all, both the timing and the spacing are clearly defined. So if you key those poses at the right times and places, you get your answer and you’re done, right?

Well, maybe. For one solution. But there are many solutions.

So the challenge is this:

Consider a variety of solutions and implementations, making an effort to resolve each solution with just those four keys in the graph editor.

(To limit obvious alternates, all solutions assume a same start/finish point, a clockwise path direction, and the use of the orthographic XY plane)

It’s an intentionally simple scenario, yet oddly enough there are still many answers to the question. Initially, I meant only to examine the answers in 2d form. But when I applied the answers to 3D effort, I realized that there was more to the matter yet again, because for each answer there were also many ways of implementing that answer in the context of rigging and animation curves (Lesson: something which seems simple will typically become complicated in ways that were not anticipated.)

When I ran the challenge through some of the most obvious answers and implementations, I was able to confirm a variety of animation rules of thumb. It was also a generally decent technical study good for solidifying some basic concepts in 3D animation. 

... more to come later! Feel free to comment if you'd like to have a go at the challenge for yourself (before I give away my own set of answers!)